3D Koch Snowflake
Because of all the political advertisements, I find myself watching more and more PBS. I am a fan of NOVA, but this week’s topic was on fractals. Fractals have always struck me as boring; but compared to 30 smear campaigns they win every time.
A segment that caught my eye was about the Koch snowflake. It looks like a snowflake but has an interesting property in that it has a finite area but an infinite perimeter. Helge von Koch described this shape in a paper in 1904 –before he had a seat of SOLIDWORKS, thus sadly his work was only in 2D.
I decided to continue the mathematician’s work in 3D. Instead of using triangles I would use tetrahedrons.
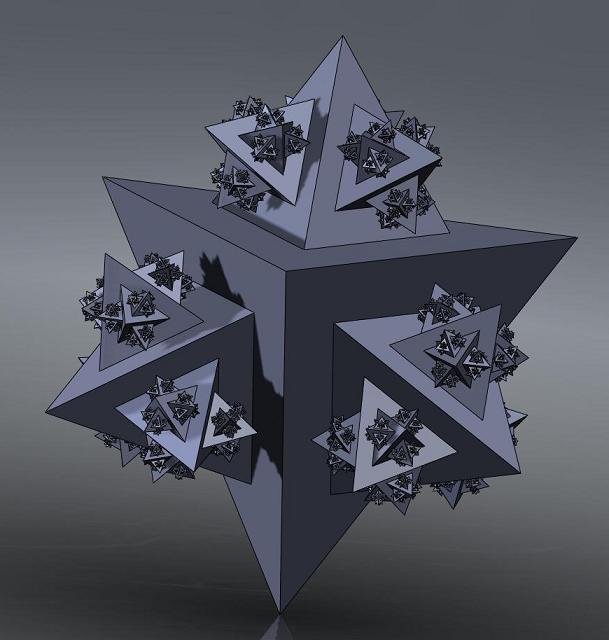
3D Koch Snowflake eDrawing [Eight iterations; 4,373 tetrahedrons]
After I completed the model, I did a search for other 3D Koch Snowflakes. Turns out mine looks nothing like any others. You might be looking at the first, most accurate representation! Who are you going to believe -a guy who sat through an entire NOVA episode, or people who have devoted their entire lives to this “science”? <sigh> I guess maybe I should not say this is a 3D Koch Snowflake, but only that it was inspired by it.
I still think fractals are for hippies, but I thought this turned out pretty cool….and perhaps a fun challenge to you SOLIDWORKS users out there, can you build it?

 Blog
Blog